Formula To Graph A Circle

Circles are simple to piece of work with in pre-calculus. A circle has one center, one radius, and a whole lot of points, but you follow slightly different steps, depending on whether y'all are graphing a circle centered at the origin or away from the origin.

The first thing you demand to know in order to graph the equation of a circle is where on a plane the center is located. The equation of a circle appears equally (ten – h)² + (y – five)^two = r ². This is called the center-radius form (or standard form) considering it gives you both pieces of information at the aforementioned time.

The h and 5 stand for the coordinates of the center of the circle being at the point (h, v), and r represents the radius. Specifically, h represents the horizontal displacement — how far to the left or to the right of the y-axis the center of the circumvolve is. The variable v represents the vertical deportation — how far above or beneath the 10-axis the middle falls.

From the center, you can count from the center r units (the radius) horizontally in both directions and vertically in both directions to get 4 different points, all equidistant from the center. Connect these four points with the all-time bend that y'all can sketch to get the graph of the circumvolve.

Graphing circles centered at the origin

The simplest circumvolve to graph is one whose eye is at the origin (0, 0). Considering both h and five are zero, they can disappear and y'all can simplify the standard circle equation to look like x ² + y ² = r ². For case, to graph the circle 10 ^two + y ² = 16, follow these steps:

1. Realize that the circumvolve is centered at the origin (no h and v) and place this signal there.

2. Calculate the radius past solving for r.

   Set r ⁱⁱ = sixteen. In this instance, you get r = 4.

3. Plot the radius points on the coordinate aeroplane.

   You count out 4 in every direction from the center (0, 0): left, right, up, and downwards.

4. Connect the dots to graph the circle using a smooth, round bend.

   

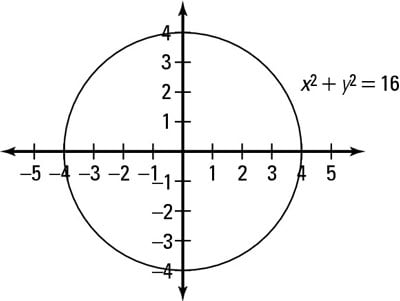
   Graphing a circumvolve centered at the origin

   The figure shows this circle on the aeroplane.

Graphing circles centered abroad from the origin

Although graphing circles at the origin is easiest, very few graphs are as straightforward and simple as those. In pre-calculus, you piece of work with transforming graphs of all unlike shapes and sizes. Fortunately, these graphs all follow the aforementioned pattern for horizontal and vertical shifts, so you don't have to retrieve many rules.

Don't forget that the coordinates of the middle of the circumvolve are of the contrary signs of the h and 5 from inside the parentheses in the equation. Because the h and five are inside the grouping symbols, this means that the shift happens opposite from what you would remember.

For example, follow these steps to graph the equation (x – iii)² + (y + 1)ⁱⁱ = 25:

1. Locate the center of the circumvolve from the equation (h, v).

   (x – 3)² means that the x-coordinate of the center is positive 3.

   (y + 1)² means that the y-coordinate of the middle is negative 1.

   Place the center of the circumvolve at (3, –1).

2. Calculate the radius by solving for r.

   Ready r ² = 25 and foursquare root both sides to get r = 5.

3. Plot the radius points on the coordinate aeroplane.

   Count five units upward, down, left, and right from the centre at (3, –ane). This footstep gives you points at (8, –1), (–2, –1), (iii, –half-dozen), and (3, 4).

4. Connect the dots to the graph of the circle with a circular, smooth curve.

   

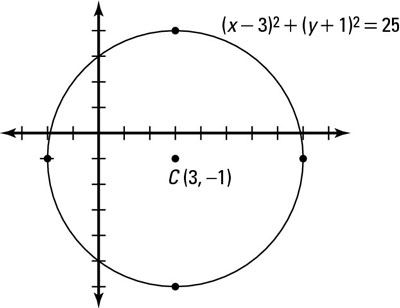
   Graphing a circle not centered at the origin

   The effigy shows a visual representation of this circumvolve.

About This Article

This article is from the volume:

• Pre-Calculus For Dummies ,

Most the book author:

Mary Jane Sterling zilch algebra, business organization calculus, geometry, and finite mathematics at Bradley Academy in Peoria, Illinois for more than thirty years. She is the writer of several For Dummies books, including Algebra Workbook For Dummies, Algebra II For Dummies, and Algebra 2 Workbook For Dummies.

This article can exist institute in the category:

• Pre-Calculus ,

Formula To Graph A Circle,

Source: https://www.dummies.com/article/academics-the-arts/math/pre-calculus/2-ways-to-graph-a-circle-167833/

Posted by: leeyoutive.blogspot.com

Share this post